An astronomical unit (AU) is exactly 149,597,870,700 meters (92,955,807 miles or 149,597,871 kilometers), according to the International Astronomical Union (IAU). This is roughly the average distance between Earth and the sun.
Astronomers use astronomical units to describe how far away objects in space are, mostly in relation to the sun or other stars. For instance, Jupiter is about 5.2 AU from the sun, according to NASA.
Because Earth's orbit around the sun is elliptical (oval-shaped), it isn't always the same distance from the sun. An astronomical unit represents a practical average, rather than a precise measurement, of our distance from the sun.
Related: How big is Earth?
Difference between AU, light-year and parsec
Astronomical units are different from some other units of measurement researchers use to describe the distances of objects in space. Another unit is the light-year, or the distance light travels in one year — around 5.88 trillion miles (9.46 trillion km), according to NASA. In contrast, 1 AU is about 8.3 light-minutes, meaning it takes light about 8.3 minutes to travel from the sun to Earth.
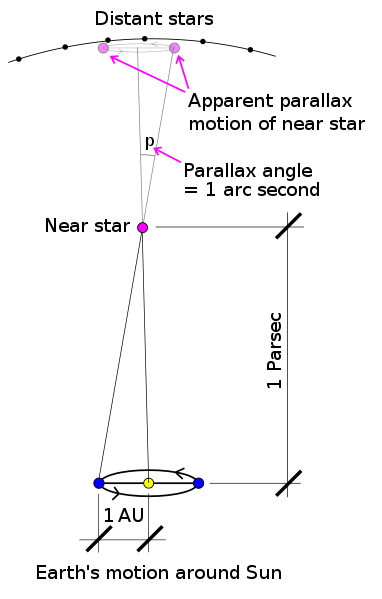
Another unit is the parsec, which is equal to about 3.26 light-years, according to NASA. The parsec is a more technical measurement that is derived from an astronomical unit and is used mainly by scientists.
Technically, a parsec is defined as the distance at which 1 AU subtends a one-arcsecond angle (see diagram to the right). The next-nearest star to the sun, Proxima Centauri, is about 1.3 parsecs, 4.25 light-years or 268,770 AU away.
Astronomical Unit FAQs
What is the length of one astronomical unit?
One astronomical unit is exactly 149,597,870,700 meters (92,955,807 miles or 149,597,871 km), as defined by the International Astronomical Union.
What are examples of astronomical units?
Earth, by definition, is 1 AU from the sun. Mercury, the closest planet to the sun, is about 0.39 AU from our star, while Neptune, the farthest planet from the sun, is 30.06 AU away from it.
What's the difference between an astronomical unit and a parsec?
An astronomical unit is the distance between Earth and the sun and measures distances on the scale of star systems. A parsec is a unit used to measure vast distances in interstellar space, such as distances between stars and galaxies, and is partially defined using an AU. One parsec is about 19 trillion miles (31 trillion km). It takes light 8.3 minutes to travel between Earth and the sun but 3.26 years to travel one parsec.
Changes to the definition
Before 2012, the definition of an astronomical unit was not defined as a constant and depended on several factors. The IAU, the international group that defines astronomical constants, decided to make the measurement simpler in August of that year.
Why the change? One reason was that the previous method of calculating an AU depended on knowing the mass of the sun, but that measurement is always changing as the sun converts its mass into energy, Nature reported. Another is related to Einstein's theory of general relativity, which posits that space-time is relative to the observer's location. The current definition addresses this problem by basing the distance on the speed of light in a vacuum, which always remains constant.
Our solar system in astronomical units
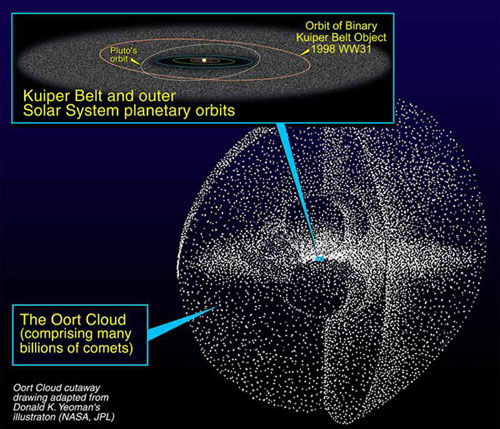
All of the bodies in the solar system — including the planets, asteroids and comets — orbit the sun at various distances. Like Earth, other planets have elliptical orbits and are not always the same distance from the sun. This is especially true for dwarf planets such as Pluto, which have highly irregular orbits. At its closest, Pluto is about 29.7 AU from the sun (closer than Neptune); at its farthest, it is about 49.3 AU away, according to NASA.
The solar system extends for thousands of astronomical units away from the sun. Mercury, the closest planet to the sun, gets as near as 29 million miles (47 million km) in its elliptical orbit, while some objects in the Oort cloud, the solar system's icy shell, are thought to lie as far as 100,000 AU to 200,000 AU from the sun.
| Planet | Distance from sun (in AU) | Header Cell - Column 2 |
|---|---|---|
| Mercury | 0.39 | Row 0 - Cell 2 |
| Venus | 0.72 | Row 1 - Cell 2 |
| Earth | 1 | Row 2 - Cell 2 |
| Mars | 1.52 | Row 3 - Cell 2 |
| Jupiter | 5.2 | Row 4 - Cell 2 |
| Saturn | 9.54 | Row 5 - Cell 2 |
| Uranus | 19.2 | Row 6 - Cell 2 |
| Neptune | 30.06 | Row 7 - Cell 2 |
| Pluto (dwarf planet, average) | 39.5 | Row 8 - Cell 2 |
Source: NASA Jet Propulsion Laboratory (see planets data and dwarf planet Pluto data)
History of the astronomical unit
The first known person to measure the distance to the sun was Greek astronomer Aristarchus of Samos, who lived from about 310 B.C. to 230 B.C. He used the phases of the moon to measure the sizes and distances of the sun and moon.
He postulated that when the half moon appears in Earth's sky, the center of our planet and the center of the moon create a line in space that forms a 90-degree angle with another line that could be drawn through space from the moon's center all the way to the sun's center. Using trigonometry, Aristarchus determined the hypotenuse of a triangle based on those two imaginary lines. The value of the hypotenuse provided the distance between the sun and Earth.
Although his measurement was imprecise, Aristarchus provided a simple understanding of the sizes and distances of the three bodies, which led him to conclude that Earth goes around the sun about 1,700 years before Nicolaus Copernicus proposed his heliocentric model of the solar system.
In 1653, astronomer Christiaan Huygens calculated the distance from Earth to the sun. Much like Aristarchus, he used the phases of Venus to find the angles in a Venus-Earth-sun triangle. His more precise measurements for what exactly constitutes an AU were possible thanks to the existence of the telescope.
Guessing (correctly, by chance) the size of Venus, Huygens was able to determine the distance from Venus to Earth. Knowing that distance, plus the angles made by the triangle, he measured the distance from Earth to the sun. However, because Huygens' method was partly guesswork and not completely scientifically grounded, he usually doesn't get the credit.
In 1672, Giovanni Cassini used a method involving parallax, or angular difference, to find the distance to Mars and, at the same time, figured out the distance to the sun. He sent a colleague, Jean Richer, to Cayenne, French Guiana (located just northwest of the modern-day Guiana Space Center, near Kourou) while he stayed in Paris. At the same time, they both took measurements of the position of Mars relative to background stars, and triangulated those measurements with the known distance between Paris and French Guiana. Once they had the distance to Mars, they could also calculate the distance from Earth to the sun. Because his methods were more scientific, Cassini usually gets the credit.
These techniques are also why astronomers continue to use the distance from Earth to the sun as a scale for interpreting the solar system.
"Expressing distances in the astronomical unit allowed astronomers to overcome the difficulty of measuring distances in some physical unit," Nicole Capitaine, an astronomer at the Paris Observatory, told Space.com in 2012. "Such a practice was useful for many years, because astronomers were not able to make distance measurements in the solar system as precisely as they could measure angles."
The sun is at the heart of the solar system. All of the bodies in the solar system — planets, asteroids, comets, etc. — revolve around it at various distances.
Mercury, the planet closest to the sun, gets as close as 29 million miles (47 million km) in its elliptical orbit, while objects in the Oort Cloud, the solar system's icy shell, are thought to lie as far as 9.3 trillion miles (15 trillion km).
Everything else falls in between. Jupiter, for example, is 5.2 AU from the sun. Neptune is 30.07 AU from the sun.
The distance to the nearest star, Proxima Centauri, is about 268,770 AU, according to NASA. However, to measure longer distances, astronomers use light-years, or the distance that light travels in a single Earth-year, which is equal to 63,239 AU. So Proxima Centauri is about 4.25 light-years away.
Astronomical Unit questions answered by an expert
Adam Riess is an astrophysicist who studies physical cosmology, measuring the universe using distance indicators such as supernovas (exploding stars) and Cepheids (pulsating stars). He also studies the expansion of the universe and was co-awarded the Nobel Prize in physics in 2011 for his role in discovering that the expansion rate of the universe is accelerating.
What sort of astronomical objects are still usually measured in AU, and when might objects be too far away for the measurement to make sense?
The AU remains the baseline for any trigonometric parallax measurements, so nearly all distances measured in the Milky Way (MW), such as from the ESA [European Space Agency] Gaia mission, are calibrated to the AU. Outside the MW, distances based on standard candles like Cepheids are also calibrated by parallax so also depend on the AU.
Geometry was a key part of how early astronomers calculated distances in space. Is it still, and how is it used?
Yes, geometry underlies all distance measurements. We just plug the physics into the geometry
How can you use objects like supernovas and Cepheids to determine distances and other astronomical phenomena?
By calibrating their luminosity, we can use their brightness and the inverse square law to determine their distances.
Additional resources
Watch a video explaining Aristarchus' approach to calculating the distance from Earth to the sun. NASA's sun fact sheet provides basic statistics about our star and its solar system exploration page offers details about solar science and missions studying the sun.
Bibliography
Brumfiel, G. "The astronomical unit gets fixed." Nature (2012). https://www.nature.com/articles/nature.2012.11416
International Astronomical Union, "Measuring the Universe," accessed Jan. 21, 2022. https://www.iau.org/public/themes/measuring/
Kish, G. "A Source Book in Geography," accessed via Google Books. Harvard University Press, 1978.
Luque, B. and Ballesteros, F. "To the Sun and beyond." Nature Physics (2019). https://www.nature.com/articles/s41567-019-0685-3
NASA Jet Propulsion Laboratory, “Solar System Sizes and Distances,” accessed Oct. 19, 2023. https://www.jpl.nasa.gov/edu/pdfs/scaless_reference.pdf
NASA Exoplanet Exploration, “What is a light-year?” accessed Oct. 19, 2023. https://exoplanets.nasa.gov/faq/26/what-is-a-light-year/
NASA Science, “Cosmic Distances,” accessed Oct. 19, 2023. https://science.nasa.gov/solar-system/cosmic-distances/
NASA Goddard Space Flight Center, “The Nearest Neighborhood Star,” accessed Oct. 19, 2023. https://imagine.gsfc.nasa.gov/features/cosmic/nearest_star_info.html
NASA Jet Propulsion Laboratory. “Planet Distance Chart,” accessed Oct. 19, 2023. https://www.jpl.nasa.gov/edu/pdfs/ssbeads_answerkey.pdf
Physics Explained. “Greek Physics: Calculating the distance to the Sun and Moon,” accessed Oct. 19, 2023. https://www.youtube.com/watch?v=urgYWNCN-RA&t=137s
Join our Space Forums to keep talking space on the latest missions, night sky and more! And if you have a news tip, correction or comment, let us know at: community@space.com.
